Study reveals the existence of the swallowtail catastrophe in non-Hermitian systems

Researchers from the Hong Kong University of Science and Technology, Xiangtan University and Southern University of Science and Technology recently unveiled a possible connection between catastrophe theory, an area of mathematics that focuses on modeling sudden changes (i.e., catastrophes) and non-Hermitian physics. Their paper, published in Nature Physics, specifically shows that a structurally rich degeneracy, known as the swallowtail catastrophe, can naturally exist in non-Hermitian systems.

“Our work was inspired by prior research that utilized homotopy theory to classify topological singularities, a technique that has been used to study defects in liquid crystals such as disclinations and dislocations,” Hongwei Jia, one of the researchers who carried out the study, told Phys.org. “Homotopy theory has also been applied in band theory to investigate non-Abelian band topology. Building on these prior works, we sought to extend the approach to understand the singularities (manifested as degeneracies called exceptional points) in non-Hermitian systems.”

Essentially, Jia, Chan and their colleagues set out to apply the concept of an eigenvector frame rotation along a loop encircling a singularity (i.e., point at which a function “jumps” or “collapses”) to non-Hermitian systems. While in other recent studies on Hermitian systems, the eigenvectors were real and orthogonal, forming the orthonormal basis of a Euclidian space, applying this eigenvector frame rotation to non-Hermitian systems poses a series of challenges.

“In these non-Hermitian systems, the eigenvectors are not orthogonal, because the orthogonal relation of eigenvectors is defined by a Minkowski-type indefinite inner product. Thus, along a closed path, the eigenvector frame not only rotates but also deforms. Particularly, when the path encounters exceptional surfaces, the eigenvectors coalesce,” Jia explained.

“This poses a serious problem because conventional homotopical loops never cut though degeneracies. But this cannot be avoided in our case, because the considered degeneracy lines are entirely embedded on exceptional surfaces. In tackling these mathematically challenging difficulties, we sought the assistance of the mathematician Yifei Zhu. This collaboration between physicists and mathematicians resulted in new insights into the topological properties of singularities in non-Hermitian systems.”

In collaboration with Zhu, Jia, Chan and their colleagues set out to explore the occurrence of degeneracies, referred to as exceptional surfaces, isolated and non-isolated singularities, in non-Hermitian systems, and the dependence of these degeneracies on symmetry. To achieve this, they tracked the zeros in the discriminant of the characteristic polynomial of Hamiltonian matrices under specific symmetries in the parameter space.

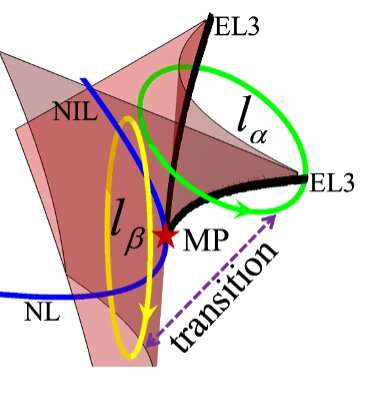
“We conducted many mathematical experiments using various tools such as math software, paper models, and 3D printing,” Jia said. “By analyzing explicit examples of Hamiltonians with specific symmetries, we discovered that the occurrence of this singularity feature is universal in non-Hermitian systems with our chosen symmetries. We also demonstrated that there can be exceptional lines of order three (EL3) at cusps of exceptional surfaces, non-defective intersection lines (NIL) where exceptional surfaces intersect transversally, and nodal lines (NL) isolated from exceptional surfaces.”

The experiments and calculations carried out by Jia and his colleagues yielded several interesting findings. Firstly, the team found that the distinct degeneracy lines they observed can always be stably connected at a single meeting point and that this unique structure is symmetry-protected.

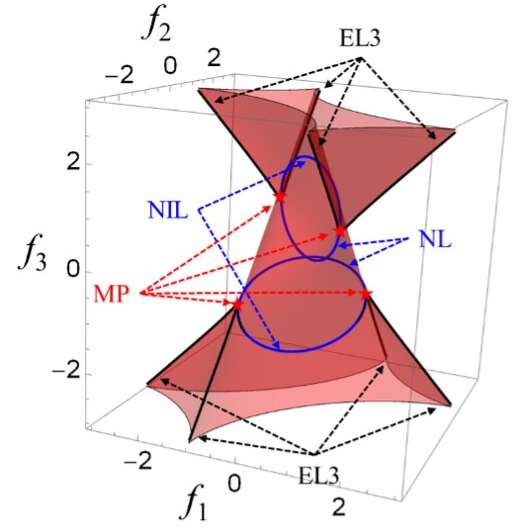
“We observed that the exceptional surfaces intersect in a swallowtail configuration, which is reminiscent of the swallowtail catastrophe in mathematics and catastrophe theory (specifically in ADE classification),” Jia said. “However, there are notable differences between our case and the swallowtail catastrophe in ADE classification, as the latter is described by examining the locus of multiple roots of a quartic polynomial whereas ours arises from the symmetries we are studying which encodes information of eigenvector evolutions.”

The recent work by this team of researchers establishes a connection between the mathematical catastrophe theory and non-Hermitian physics, two areas of study that were previously perceived as unrelated. Using homotopical methods, the team tried to gain a topological understanding of non-isolated singularities in non-Hermitian systems.

Jia and his colleagues ultimately unveiled several interesting new transitions that occur within the swallowtail structure of the catastrophe they observed. Notably, these counter-intuitive transition phenomena are protected in ways that previous research had not yet identified.

“This work is the result of a collaboration between myself and fellow physicist Che Ting Chan and mathematician Yifei Zhu,” Jia said. “The introduction of intersection homotopy theory by Yifei is crucial in solving the problem. We combined our theory of eigen-frame deformation and rotation with intersection homotopy, and successfully demonstrated that the interesting transition phenomenon in a swallowtail is topologically protected. We believe there may be other interesting physical phenomena to be discovered in these platforms. Our complementary knowledge will enable us to further explore this uncharted area of research.”

The swallowtail catastrophe that Jia and his colleagues observed in non-Hermitian bands is an entirely new type of topological gapless phase. Further examinations of this phase could potentially unveil new physical phenomena and effects. The researchers are now conducting studies focusing on two intriguing phenomena, the first of which is the bulk–edge correspondence in this new type of gapless phase.

“We are exploring whether the gapless phases inherent in a swallowtail structure can also support topological edge states,” Jia said. “The second phenomenon we are exploring is the unconventional bulk-Fermi arc that links the pair of exceptional lines of order three at paired cusps.”

In addition to informing future physics studies, the findings gathered by this team of researchers could lead to new research in the field of mathematics. Jia and his colleagues feel that the mathematical component of their work is still ad hoc and incomplete, and they plan to further develop it in their next works.

“In theory, despite the objects of study can already be formulated purely mathematically (in ADE classification), this formulation only gives the apparently comparable structure, while the underlying characteristics are quite different from the current case,” Jia explained. “For example, the meeting point of the swallowtail in ADE classification is an exceptional point of order four, but that of the current swallowtail is a three-fold degeneracy affording two linearly independent eigenstates.

“It would be a real challenge and opportunity to get hold of mathematically systematic, physically meaningful, and experimentally realizable structures underneath this tip of an iceberg. We also believe that the algebraic method, intersection homotopy/homology, should be further developed, because it is a powerful tool for understanding such non-isolated singularities both in physics and mathematics.”

© 2023 Science X Network